To understand how to study the
relationship between two variables when both are quantitative, one needs a
basic understanding of a correlation coefficient.
Correlation is the relationship between
two or more paired variables or two or more sets of data. The degree of
relationship is measured and represented by the coefficient of correlation.
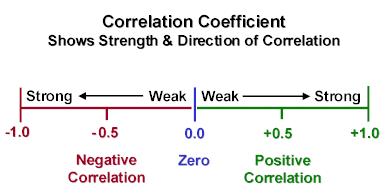
It is a numerical index that provides
information about the strength and direction of the relationship between two
variables. It provides information about how two variables are associated.
More specifically, a correlation
coefficient is a number that can range from -1 to 1, with zero standing for no
correlation at all.
Positive
Correlation:
If the number is greater than zero,
there is a positive correlation. (A positive correlation is present when scores
on two variables tend to move in the same direction).
Negative
Correlation:
If the number is less than zero, there
is a negative correlation. (A negative correlation is present when scores on
two variables tend to move in opposite directions—as one variable goes up, the
other tends to go down and vice versa)
No
Correlation:
If the number is equal to zero, then
there is no correlation between the two variables being correlated.
Perfect
Correlation:
If the number is equal to +1 or equal
to -1, the correlation is called perfect; that is, it is as strong as possible.
Data
analysis for Correlation Research:
Pearson product-moment—when you have
two interval or ratio scale variables
Spearman—when variables are at least
ordinal
Multiple correlation—relationship
between one variable and a set of variables
Canonical correlation—relationship
between two sets of variables
Partial correlation—correlation of one
variable with another after statistically removing the effects of a third
variable
Coefficient of determination—squared
correlation—explains the variability in the first variable (proportion of
variance accounted for)
Spurious correlation—significant
correlation between two variables that is likely due to coincidence, i.e.,
there is no obvious reason why the two variables should be correlated but they
are.